Third Law of planetary motion, Imagine a cosmic ballet, where planets gracefully pirouette around the Sun. Their movements, though seemingly random, are governed by a set of precise rules. One such rule, Kepler’s Third Law of planetary motion, unveils the connection between a planet’s distance from the Sun and its orbital period.
Tycho Brahe’s precise astronomical observations paved the way for Johannes Kepler’s formulation of the Third Law of Planetary Motion, which describes the relationship between a planet’s orbital period and its distance from the Sun. While Brahe initially supported the idea of circular orbits, his extensive data allowed Kepler to demonstrate that planets move in elliptical orbits rather than perfect circles.
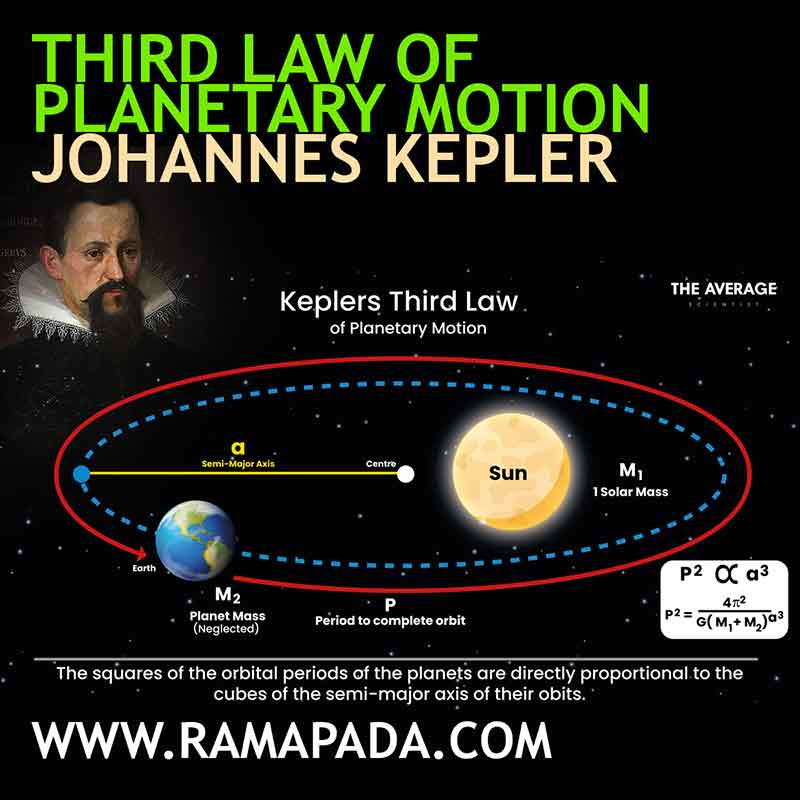
Kepler’s Third Law, also known as the Law of Harmonies, states that the square of a planet’s orbital period is proportional to the cube of the semi-major axis of its orbit. This discovery refined our understanding of celestial mechanics, showing that planets revolve around the Sun in predictable patterns. Additionally, Kepler’s Second Law—which states that a planet sweeps out equal areas in equal times—helped explain variations in planetary speed. Brahe’s meticulous records provided the empirical foundation for Kepler’s groundbreaking laws, transforming our comprehension of planetary motion
The Law
Developed by Johannes Kepler in the early 17th century, this law describes the harmonious relationship between a planet’s orbital distance and the time it takes to complete one revolution around the Sun. Here’s the gist:
- The square of a planet’s orbital period (P) is proportional to the cube of the semi-major axis (a) of its orbit.
In simpler terms, planets farther from the Sun take considerably longer to complete one orbit compared to their closer counterparts.
Let’s unpack this further. The semi-major axis represents the average distance between a planet and the Sun in its elliptical orbit. As the distance (a) increases, the cube of this distance (a^3) grows much faster. The law states that this rapid growth is balanced by the square of the orbital period (P^2). So, a planet twice as far from the Sun will have an orbital period that’s not just double, but roughly four times longer.
Implications of the Law
Kepler’s Third Law of planetary motion has profound implications for our understanding of the solar system. It allows us to:
- Estimate orbital periods: Knowing the distance of a planet from the Sun, we can calculate its approximate orbital period. This was particularly useful before the era of space exploration when directly measuring orbital periods was challenging.
- Compare planetary systems: The law applies not only to our solar system but also to planets orbiting other stars (exoplanets). By observing the exoplanet’s wobble on its host star (a technique called radial velocity), astronomers can estimate its orbital period. This, coupled with the star’s distance, can hint at the exoplanet’s potential habitability.
A Stepping Stone to Gravity
While Kepler didn’t understand the force governing planetary motion, his Third Law laid the groundwork for Isaac Newton’s law of universal gravitation. By explaining the relationship between distance and orbital period, Kepler’s law provided a crucial piece of the puzzle that ultimately led to our understanding of gravity and celestial mechanics.
Kepler’s Third Law of planetary motion, a testament to scientific observation and deduction, continues to be a cornerstone in our exploration of the cosmos. It elegantly encodes the rhythm of the planets, revealing the order within the celestial dance.